Type – 1 Choose the most appropriate option (a, b, c or d). Q 1. ABCDEF is a regular hexagon where centre O is the origin. If the position vectors of A and B are → → → → i − j + 2k → and → → → 2i + j + k → BC respectively then → → i − j + 2k → is equal to → → − i + j − 2k (a) → → 3 i + 3 j− 4k (b) (c) (d) none of these → → → → → → i + j,2 i − j + 4 k Q 2. The position vectors of two vertices and the centroid of a triangle are k and respectively. The position vector of the third vertex of the triangle is → → → → −3 i + 2 k 2→ k 3 → → i+ → 3 i − 2k (a) (b) (c) → (d) none of these → → → → i + 2 j, +3 k, − i − j + 8 k Q 3. Let the position vectors of the points A, B, C be → → −4 i + 4 j,6 k and respectively. Then the ABC is (a) right angled (b) equilateral (c) isosceles (d) none of these → → → → a,b,c Q 4. → a+ b are three vectors of which every pair is noncollinear. If the vector → → c with → → a+ b+ c a and → and are collinear respectively then is → → → a,b,c (a) a unit vector → → → (b) the null vector → → → → → → → → (c) equally inclined to → → r = 3 i + 2 j − 5 k,a = 2 i − j + k,b = i + 3 j − 2 k Q 5. If → → (d) none of these → → c = 2 i + j− 3k and s → → such that then λ µ, ,v arein AP 2 (a) (b) , , v are in AP → (c) , , v are in HP → → → → → Q 6. The position vectors of three points are noncoplanar vectors. The are collinear when (d) , , v are in GP → 2 a − b + 3 c,a − 2 b + λ c → → → → µ a− 5 b and → r = λ a+ µ b+ v c a,b,c where are

9 4 9 λ = − ,µ = 2 4 (a) (b) → → → → → → → (c) → → a = i + j + k,b = 4 i + 3 j + 4 k Q 7. 9 , µ = −2 4 λ= If then → → (d) none of these → → c = i + α j + βk and | c |= 3 are linearly dependent vectors and (a) = 1, = – 1 (b) = 1, = 1 (c) = – 1, = 1 → → → OA = a Q 8. (d) = 1, = 1 → OB = b Let and . A vector along one of the bisectors of the angle AOB is → → a → → → (a) Q 9. → → a−b a+ b + |a| (b) b → |b| (c) (d) none of these A vector has components 2p and 1 with respect to a rectangular Cartesian system. The axes are rotated through an angle about the origin in the anticlockwise sense. If the vector has components p + 1 and 1 with respect to the new system then 1 3 1 3 (a) p = 1, – → (c) p = – 1 , (d) p = 1, – 1 → a Q 10. (b) p = 0 → b If and → a are two vectors of magnitude inclined at an angle 60 then the angle between → a+ b is (a) 30 (b) 60 → → → (c) 45 → → | a | = | b | =| a − b | = 1 Q 11. Let → a Then the angle between π 6 b and π 3 (a) (d) none of these is π 4 (b) π 2 (c) (d) → → → → → i + j, j + k k + i Q 12. A vector of magnitude 4 which is equally inclined to the vectors 4 3 → → 4 → ( i − j − k) 3 (a) → (b) → → → a+b= 2 i Q 13. If → → → → 4 → ( i + j − k) 3 → → is → ( i + j + k) (c) → → 2a − b = i − j and (d) none of these → a then cosine of the angle between b and is and
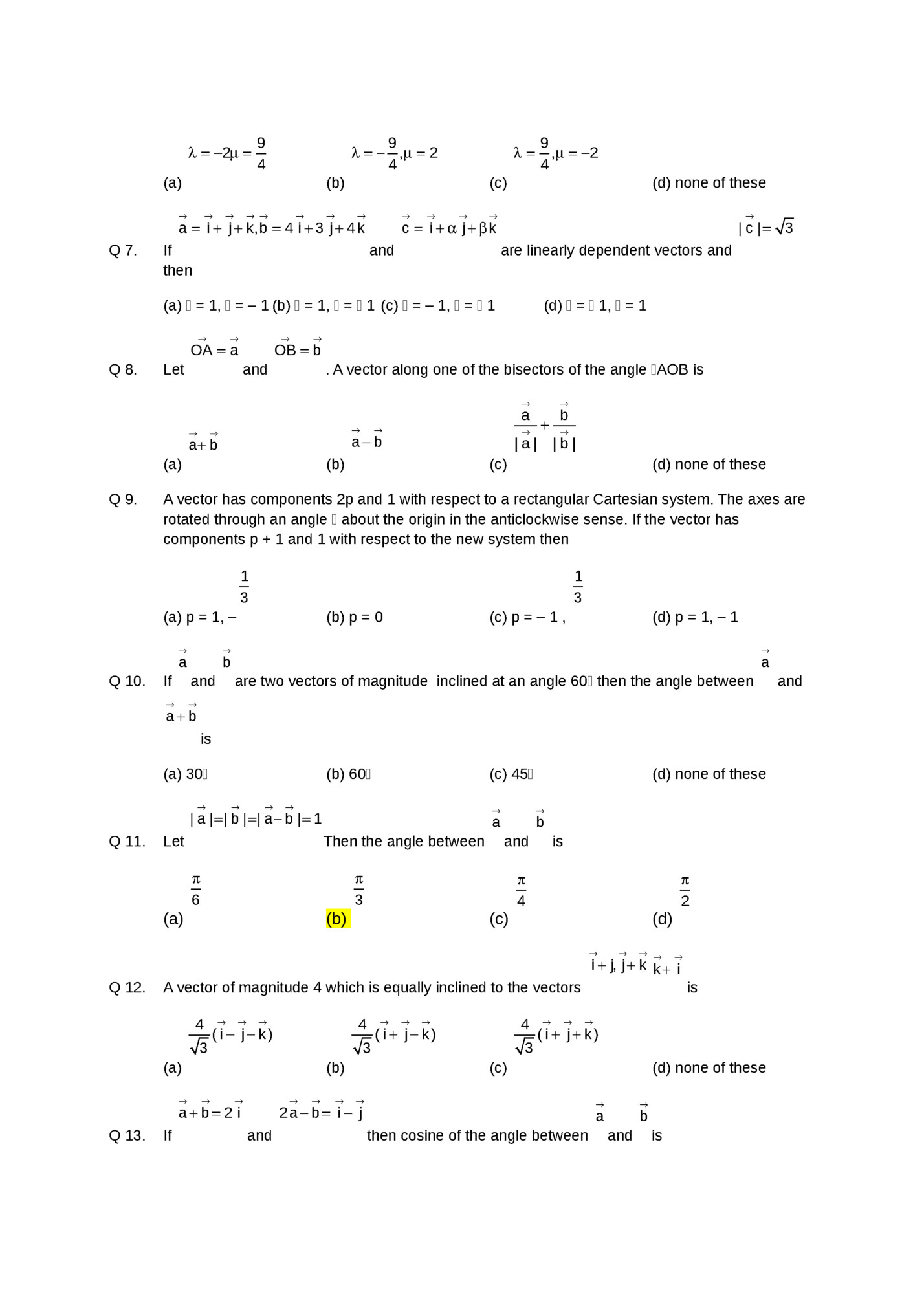
4 5 4 5 cos−1 (a) (b) → → → Let → → → → → (d) none of these → → a ⊥ (b + c),b ⊥ (c + a) and 6 (a) these 3 5 (c) | a | = 1,| b |= 2, | c |= 3, Q 14. cos−1 → → → c ⊥ (a + b) and → → | a+ b + c | . Then is 14 (b) 6 → → → → → → (c) (d) none of → → (a. i ) i + (a. j ) j + (a.k) Q 15. is equal to → → → → i+ j+k (a) Q 16. → a 3a (b) (c) If a, b, are unit vectors such that a + b is also a unit vector then the angle between the vectors a and b is π 6 π 3 π 4 (a) (b) → → → → → → → → → If → a is → → i− j (b) → → → → → → j i (a) (d) → then → 2π 3 (c) a. i = a.( i + j ) = a.( i + j + k) = 1 Q 17. (d) none of these k (c) (d) → (a. i )2 + (a. j )2 + (a + k )2 Q 18. is equal to → → (a) (b) 3 → → → → → | a.( i + j + k ) |2 a → → (c) k (d) → | a + b |2 − | a − b |2 Q 19. is equal to → → → → 4 | a.b | 4 a.b (a) Q 20. (b) 0 (c) If a,b,c are the pth, qth, rth terms of an HP and → → → i j k µ = (q − r) i + (r − p) j + (p − q)k, v + + a b c → then → → → → (d) none of these

→ → µ.v (a) µ.v are parallel vectors (b) → → → µ.v = 1 → → → a+ b ⊥ a If → → and → → then → → → → → (2 a + b) ⊥ b (a) → → → → → → → 1 → (− j + k ) 2 → 3 → → 1 → ( − i − j + k) → → → → → → → → → → 3 → → → ( i − j − k) (d) → λ, µ, v (a) 1 → then . Then → → → → is perpendicular to c → and → → a v = c x(a + b) Let λ+ µ = v → 5 → → ( i −2 j) (c) λ = a x (b + c), µ = b x(c + a) → c be coplanar. If (b) → → c and a unit vector (a) → (2 a + b) ⊥ a (d) → Let = → → (c) a = 2 i + j + k,b = i + 2 j − k 1 → (2 a − b) ⊥ b (b) → (b) → → λ+ v = 2 µ are coplanar → → → → → (c) → (d) none of these → → Let be three unit vectors such that respectively then cos + cos is equal to → → → | a + b + c | = 1 a ⊥ b. a,b,c Q 24. → | b |= 2 | a | (2 a + b) || b Q 23. → (d) → Q 22. → µxv = i + j+ k (c) Q 21. are orthogonal vectors a,b c If makes angles , with 3 2 (a) (b) 1 (c) – 1 (d) none of these π 3 → →→ a,b c Q 25. If are three vectors of equal magnitude and the angle between each pair of vectors is → → → → | a+ b + c | = 6 such that |a| then is equal to 1 6 3 (a) 2 (b) – 1 (c) 1 (d)
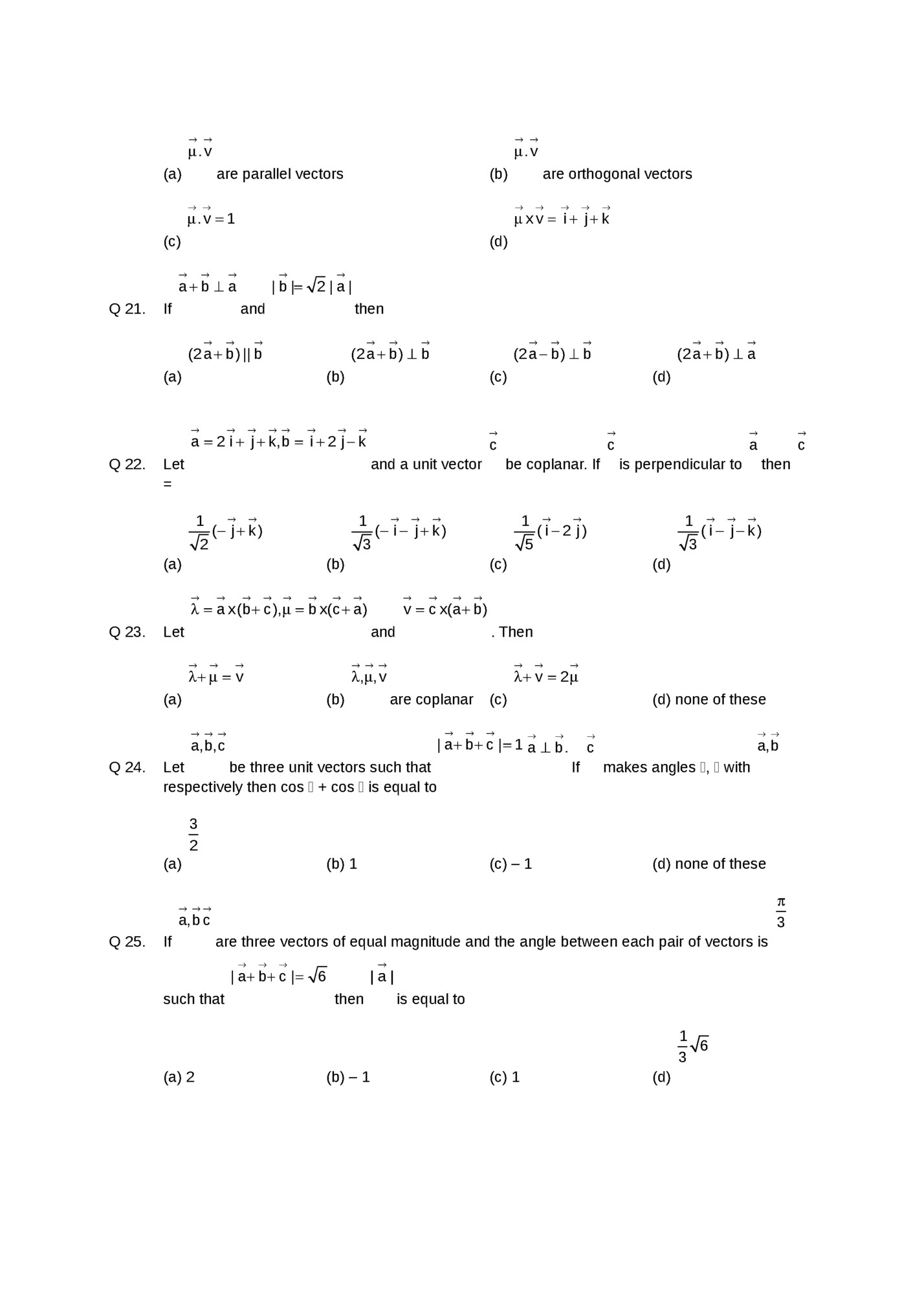
→ → | a | = 5,| a − b | = 8 Q 26. If → | a+b | |b| and = 10 then is 57 (a) 1 (b) → If (d) none of these α 2 → a Q 27. (c) 3 b and are unit vectors and is the angle between them then cos 1 → → | a+ b | 2 1 → → | a−b | 2 (a) → → → → | a+ b | (b) → is (c) (d) none of these → | a + b | = | a− b | Q 28. If then → → → (a) → → a= i+ → i Two vectors (c) → b= 3 → | a |=| b | (b) → Q 29. → a⊥ b a|| b → i (d) none of these → + j 3 and are (a) perpendicular to each other (b) parallel to each other π 3 π 6 (c) inclined to each other at an angle → → → → → → → → → a = 2 i − j + k,b = i + 3 j − k Q 30. Let → → → and → → → → b . Avector in the plane if c and whose → a projection on → c = i + j − 2k → a has the magnitude → is → → 2 i + 3 j− 3k (a) → (d) inclined to each other at an angle → → → 2 i + 3 j+ 3k → → −2 i − j + 5 k (b) (c) (d) 2 i + j+ 5k → → → → → → AB.BC + BC.CA + CA .AB Q 31. ABC is an equilateral triangle of side a. The value of is equal to 3a2 2 (a) these − (b) 3a2 (c) 3a2 2 (d) none of
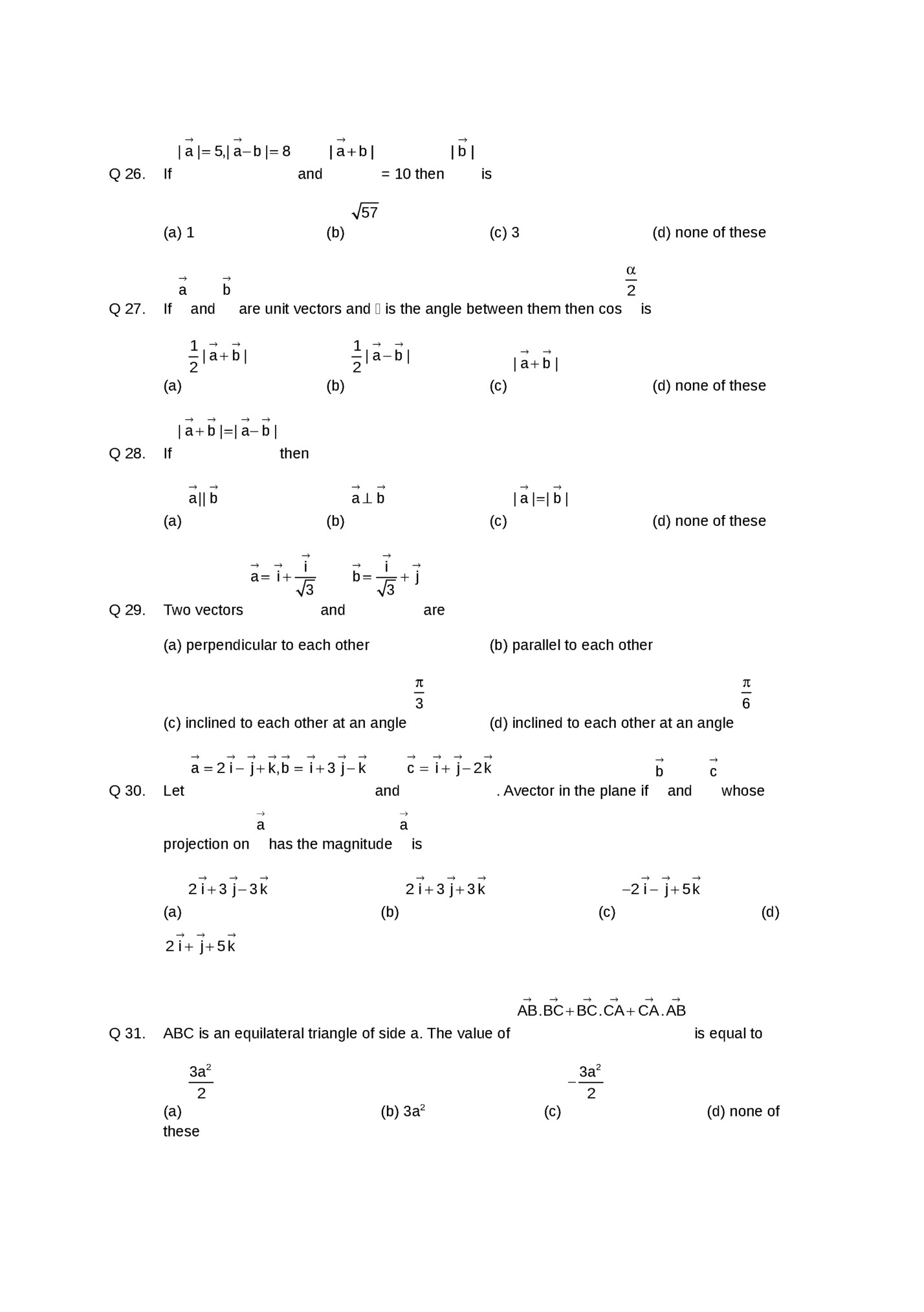
r → → → → → → → a = i + j,b = 2 j − k Q 32. If → → → → → → → r x a = b x a, r x b = a x b and 1 → 11 → 1 → → 11 → is equal to 1 → ( i − 3 j + k) → → → ( i − j + k) 11 (b) → |r| then ( i + 3 j− k) (a) → → (c) (d) none of these → → (a x b)2 + (a.b)2 Q 33. is equal to → → → (a) 0 → (| a | + | b |)2 | a |2 | b |2 (b) (c) (d) 1 → → p, q Q 34. If are two noncollinear and nonzero vector such that → → → → (b − c)p x q + (c − a)p + (a − b) q = 0, where a,b,c are the lengths of the sides of a triangle, then the triangle is (a) right angled (b) obtuse angled → → → → If → → → → (d) isosceles → → (a + b).c = (a − b).c = 0 a,b,c Q 35. (c) equilateral are any three vectors such that → b (b) (c) → (d) none of these → → → → a = i + j+ k Q 36. is → a (a) → then → 0 → (a x b) x c Then nit vector perpendicular to both the vectors → → → b = 2 i − j+ 3k and and making an → k acute angle with the vector − 1 26 → → is 1 → (4 i − j − 3 k ) (a) → 26 1 → 26 (b) → → → → → → → Let → → (a) 1 → → → → c = λ i + j (2λ − 1)k c . If is parallel to the plane of the → a vectors → → (d) none of these and → → (4 i − j + 3 k ) (c) a = i − 2 j + 3 k,b = 2 i + 3 j − k Q 37. → (4 i − j − 3 k) b and then is (b) 0 (c) – 1 (d) 2
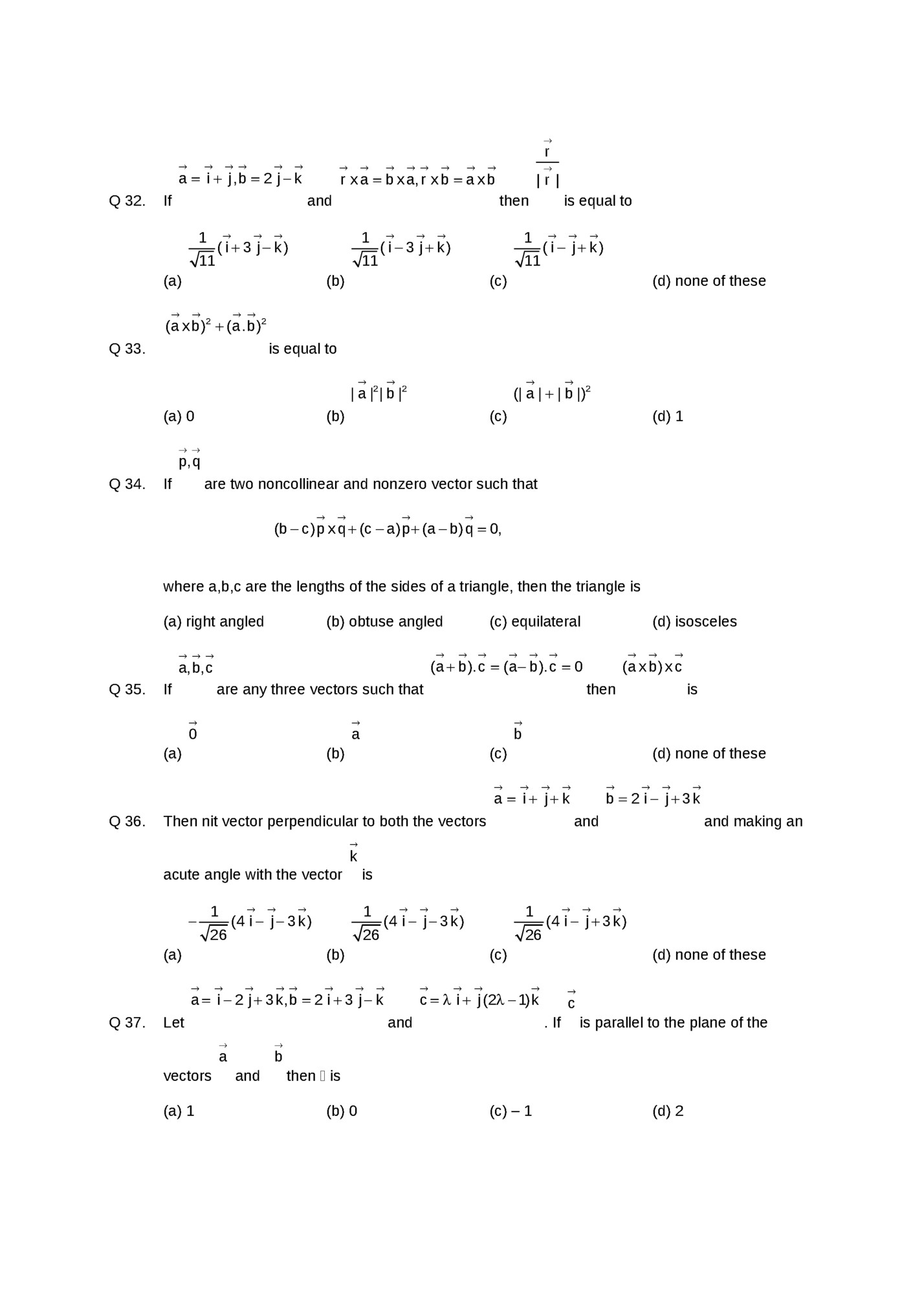
→ a Q 38. → b Let be a unit vector perpendicular to unit vectors → → c and b and if the angle between and be → bxc then is → → (a) sin α a (b) → → → a.b = 0 Q 39. → cos ec α a cos α a If → (d) none of these → axb = 0 and → (c) then → → a || b → → a⊥b (a) → → → a = b or = b = 0 (b) (c) (d) none of these → → → → 3 i + j − 2k Q 40. The area of the parallelogram whose diagonals represent the vectors is 10 3 (b) → and 5 3 (a) → → → → → → → → (c) 8 → → → (d) 4 → ( r . i )( r x i ) + ( r . j )( r x j ) + ( r .k)( r x k) Q 41. is equal to → → 3r r (a) (b) → → → → → → (c) 8 → → a = i + j + k,c = j − k Q 42. Let . If 1 → → → (5 i + 2 j + 2 k ) 3 (a) Q 43. (d) none of these → → → → axb = c is a vector satisfying 1 → → → (5 i − 2 j − 2 k ) 3 and → → → a.b = 3 b then is → 3 i − j−k (b) (c) (d) none of these A unit vector perpendicular to the plane passing through the points whose position vector are → → → → → → i − j + 2k− 2 i − k → 2i+k and → → is 1 → 2 i + j+ k 6 (a) → → → → 1 → 6 → a.b ≠ 0 and v, where → r . Then → → ( i + 2 j + k) (c) → → r xa = b xa Let → (2 i + j + k ) (b) → Q 44. → b is equal to → i − 3 j+ 4k (d) none of these

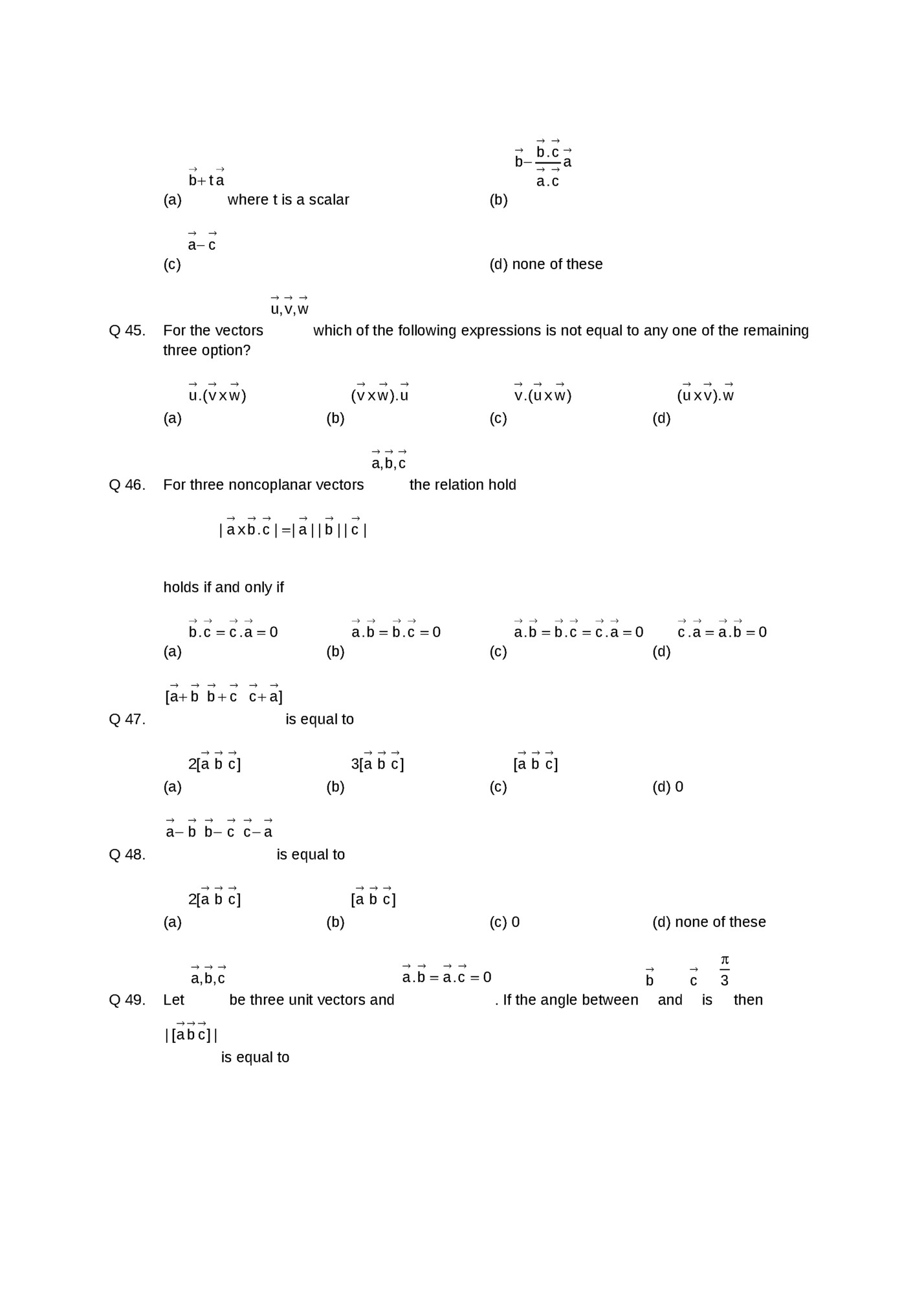
→ → b.c → b− → → a a.c → b+ t a (a) where t is a scalar → (b) → a− c (c) (d) none of these → → → u, v,w Q 45. For the vectors three option? → → which of the following expressions is not equal to any one of the remaining → → u.(v x w) → → → (v x w).u (a) → → → v .(u x w) (b) → → (u x v).w (c) (d) → → → a,b,c Q 46. For three noncoplanar vectors → → → → → the relation hold → | a x b.c | = | a | | b | | c | holds if and only if → → → → → → b.c = c .a = 0 (a) → → → → → a.b = b.c = 0 (b) → → → → → → → → → → a.b = b.c = c .a = 0 (c) → → c .a = a.b = 0 (d) → [a + b b + c c + a] Q 47. is equal to → → → → → → 2[a b c] (a) → → → → 3[a b c] [a b c] (b) → → → → (c) (d) 0 (c) 0 (d) none of these → a− b b− c c − a Q 48. is equal to → → → → → → 2[a b c] (a) [a b c] (b) → → → → → a,b,c Q 49. Let → → → a.b = a.c = 0 be three unit vectors and →→→ | [a b c] | is equal to b . If the angle between π 3 → c and is then
2 1 2 (a) (b) (c) 1 (d) none of these → → → p, q, r Q 50. Let a,b,c, be three distinct positive real numbers. If → → → → → → → → p = a i − a j + b k, q = i + k → → r = c i + c j+ bk and (a) then AM of a,c lie in a plane, where → , then b us (b) then GM if a,c → (c) sthem HM of a,c (d) equal to 0 → → [a b c] Q 51. Which of the following is not equal to s → → → → a.b x c → → → → c x a.b (a) → → → → [a b c] If (c) → a.b x c → → → → + → b.c x a → c x a.b → → → → + a x b.c (d) → → → → b x c .a (b) 1 (c) 0 (d) none of these → → → → → → p, q, r a,b,c are noncoplanar vectors and → → p= → bxc →→→ → → → → → → → are defined as → c xa → ,q = [b c a] → → c .a x b is equal to (a) 3 → → → c .a x b = 1 then Q 53. → b.a x c (b) → → Q 52. ? →→→ → ,r = [c a b] → axb → →→→ [a b c] → (a + b).p + (b + c). q+ (c + a). r is equal to (a) 0 (b) 1 (c) 2 (d) 3 → → → a,b,c Q 54. If are three noncoplanar vectors represented by concurrent edges of a parallelepiped of volume 4 then → → → → → → → → → → → (a + b).(b x c) + (b xc).(c x a) + (c + a).(a x b) is equal to (a) 12 (b) 4 (c) 12 (d) 0

Fleepit Digital © 2021